Next: Iso-neutral diffusion and eddy Up: Discrete Invariants of the Previous: Conservation Properties on Vertical Contents Index
The numerical schemes used for tracer subgridscale physics are written such
that the heat and salt contents are conserved (equations in flux form).
Since a flux form is used to compute the temperature and salinity,
the quadratic form of these quantities (![]() their variance) globally tends to diminish.
As for the advection term, there is conservation of mass only if the Equation Of Seawater is linear.
their variance) globally tends to diminish.
As for the advection term, there is conservation of mass only if the Equation Of Seawater is linear.
constraint of conservation of tracers:
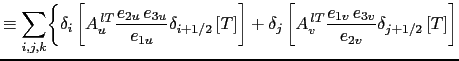 |
||
![$\displaystyle \equiv \sum\limits_{i,j,k} \biggl\{ \biggr. \delta_i \left[ A_u^{...
...v^{ lT} \frac{e_{1v} e_{3v}} {e_{2v}} \delta_{j+1/2} \left[ T \right] \right]$](img1707.png) |
||
![$\displaystyle \qquad \qquad \qquad \qquad \qquad \qquad \quad \;\;\; + \delta_k...
..._{2t}} {e_{3t}} \delta_{k+1/2} \left[ T \right] \right] \biggr\} \quad \equiv 0$](img1708.png) |
In fact, this property simply results from the flux form of the operator.
constraint on the dissipation of tracer variance:
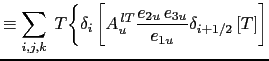 |
|||||
![$\displaystyle \equiv \sum\limits_{i,j,k} \; T \biggl\{ \biggr. \delta_i \left[ A_u^{ lT} \frac{e_{2u} e_{3u}} {e_{1u}} \delta_{i+1/2} \left[T\right] \right]$](img1711.png) |
|||||
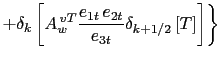 |
![$\displaystyle + \delta_k \left[A_w^{ vT}\frac{e_{1t} e_{2t}} {e_{3t}}\delta_{k+1/2}\left[T\right]\right] \biggr\}$](img1714.png) |
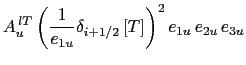 |
![$\displaystyle A_u^{ lT} \left( \frac{1} {e_{1u}} \delta_{i+1/2} \left[ T \right] \right)^2 e_{1u} e_{2u} e_{3u}$](img1716.png) |
|||
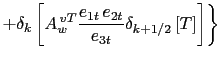 |
Gurvan Madec and the NEMO Team
NEMO European Consortium2017-02-17